Physics 11th System of particles and Collision

• 
  

  System of particles and Collision

  
  
  
  

  (1) Introduction

  
  

  
  
  • Untill now we have focused on describing motion of a single particle in one, two or three dimensions. By particle we mean to say that it has a size negligible in comparison to the path ravelled by it.
  • When we studied Law's of motion we have applied them even to the bodies having finite size imagining that motion of such bodies can be described in terms of motion of particles.
  • While doing so we have ignored the the internal structure of such bodies. Any real body we encounter in our daily life has a finite size and idealised model of particle is inadequat when we deal with motion of real bodies of finite size .
  • Real bodies of finite size can also be regarded as the system of particles. While studying system of particles we will not concentrate on each and every particle of the system instead we will consider the motion of system as a whole.
  • Large number of problens involving extended bodies or real bodies of finite size can be solve dby considering them asRigid Bodies. We define rigid body as a body having definite and unchanging shape. 
  • A rigid body is a rigid assembly of particles with fixed inter-particle distances. In actual bodies deformations do occur but we neglect them for the sake of simplicity.
  • In this chapter we will study about centre of mass of system of particles, motion of centre of mass and about collisions.

  
  
  

  (2) Centre of mass

  
  

  
  
  • Consider a body consisting of large number of particles whose mass is equal to the total mass of all the particles. When such a body undergoes a translational motion the displacement is produced in each and every particle of the body with respect to their original position.
  • If this body is executing motion under the effect of some external forces acting on it then it has been found that there is a point in the system , where if whole mass of the system is supposed to be concentrated and the nature the motion executed by the system remains unaltered when force acting on the system is directly applied to this point. Such a point of the system is called centre of mass of the system.
  • Hence for any system Centre of mass is the point where whole mass of the system can be supposed to be concentrated and motion of the system can be defined in terms of the centre of mass.
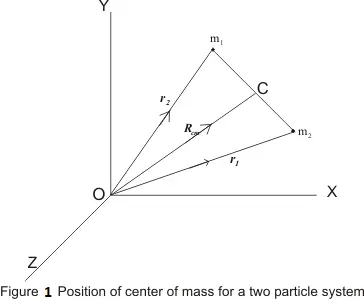
  • Consider a stationary frame of refrance where a body of mass M is situated. This body is made up of n number of particles. Let m_i be the mass and r_i be the pisition vector of i'th particle of the body.
  • Let C be any point in the body whose position vector with respect to origin O of the frame of refrance is R_c and position vector of point C w.r.t. i'th particle is r_ci as shown below in the figure.
    
    
    
  • From triangle OCP
    r_i=R_c+r_ci                               (1)
    multiplying both sides of equation 1 bt m_i we get
    m_ir_i=m_iR_c+m_ir_ci
    taking summation of above equation for n particles we get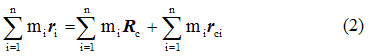
    
    
    If for a body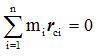
    
    
    then point C is known as the centre of mass of the body.
  • Hence a point in a body w.r.t. which the sum of the product of mass of the particle and their position vector is equal to zero is equal to zero is known as centre of mass of the body.

  
  

  (3) Position of centre of mass

  
  (i) Two particle system
  

  • Consider a system made up of two particles whose mass are m₁ and m₂ and their respective position vectors w.r.t. origin O be r₁ and r₂ and R_cm be the position vector of centre of mass of the system as shown below in the figure. So from equation 2
    
    
     
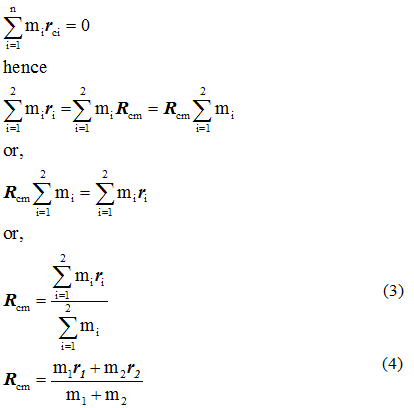
  • If M=m₁+m₂=total mass of the system , then
    
     

  (ii) Many particle system
  

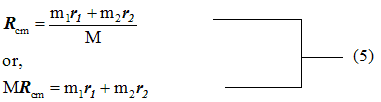
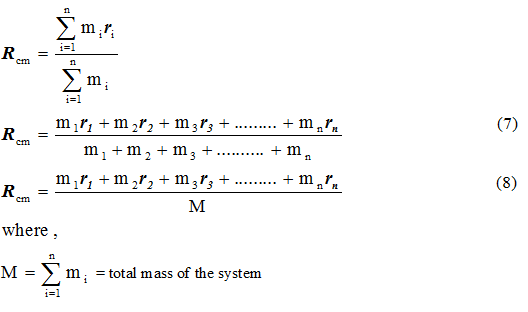
  • Consider a many particle system made up of number of particles as shown below in the figure. Let m₁ , m₂ , m₃ , . . . . . . . . . . . . . , m_n be the masses of the particles of system and their respective position vectors w.r.t. origin are r₁ , r₂ ,r₃ , . . . . . . . . . . . . . . . . . , r_n.
    
    
    
    
    Also position vector of centre of mass of the system w.r.t. origin of the refance frame be R_cm then from equation 2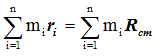
    
     
  • Because of the definition of centre of mass
    
    

  
  

  
  

  (4) Position vector of centre of mass in terms of co-ordinate components

  
  

  
  
  • Let in a system of many particles the co-ordinates of centre of mass of the system be (X_cm,Y_cm,Z_cm) then position vector of centre of mass would be
    R_cm=X_cmi+Y_cmj+Z_cmk                    (9)
    and position vectors of various particles would be
    EQ
  • Putting the values from equation 9 and 10 in equation 6, 7 and 8 we get
    
     
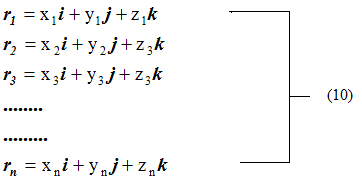
  • If in any system there are infinite particles of point mass and are distributed continously also if the distance between them is infinitesimally small then summation in equations 6, 11, 12 and 13 can be replaced by integration. If r is the position vector of very small particle of mass dm of the system then position vector of centre of the system would be
    
    
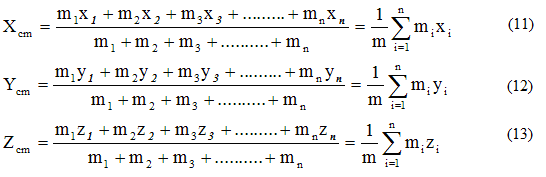
    and the value of its co-ordinates would be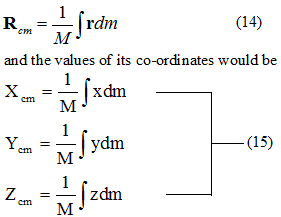
    
     
  • If ρ is the density of the system then dm=ρdV where dV is the very small volume element of the system then,
    
     
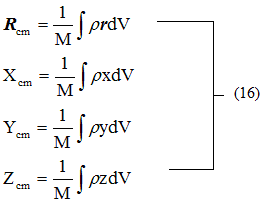
  • The centre of mass of a homogeneous body (body having uniform distribution of mass) must concide with the geometrical centre of the body. In other words we can say that if the homogeneous body has a point , a line or plane of symmetry , then its centre of mass must lie at this point , line or plane of symmetry.
  • The centre of mass of irregular bodies and shape can be found using equations 14, 15, 16.

  
  
  

  (5) Motion of centre of mass

  
  

  
  
  • Differentiating equation 6 we get
    
    
    
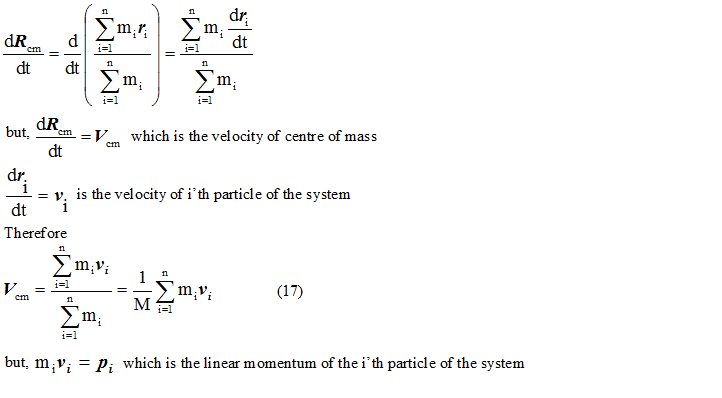
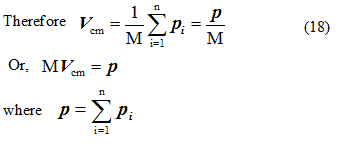
    p is the vector sum of linear momentum of various particles of the system or it is the total linear momentum of the system.
  • If no external force is acting on the system then its linear momentum remains constant. Hence in absence of external force
    
     
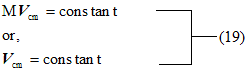
  • In the absence of external force velocity of centre of mass of the system remains constant or we can say that centre of mass moves with the constant velocity in absence of external force.
  • Hence from equation 18 we came to know that the total linear momentum of the system is equal to the product of the total mass of the system and the velocity of the centre of mass of the system which remains constant.
  • Thus in the absence of the external force it is not necessary that momentum of individual particles of system like p₁ , p₂ , p₃. . . . . . . . . . . p_n etc. remains constant but their vector sum always remains constant.

  
  

  
  

  
  

  (6) Acceleration of centre of mass

  
  

  
  
  • Differentiating equation 7 we get
    
     
  • If m_ia_i = F_i which is the force acting on the i'th particle of the system then
    
     
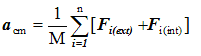
  • Net force acting on the i'th is
    F_i=F_i(ext)+F_i(int)
    Here internal force is produced due to the mutual interaction between the particles of the system. Therefore, from Newton's third law of motion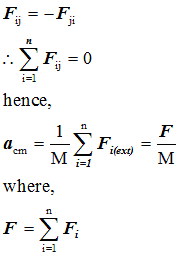
    
    is the total external force acting on the system since internal force on the system because of mutual interaction between the particles of the system become equal to zero because of the action reaction law.
  • Hence from equation 23 it is clear that the centre of mass of the system of particles moves a if the whole mass of the system were concentrated at it. This result holds whether the system is a rigid body with particles in fixed position or system of particles with internal motions.

  
  

  
  

  (7) Kinetic energy of the system of particles

  
  

  
  
  • Let there are n number of particles in a n particle system and these particles possess some motion. The motion of the i'th particle of this system would depend on the external force F_i acting on it. Let at any time if the velocity of i'th particle be v_i then its kinetic energy would be
     
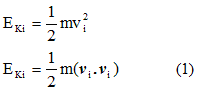
  • Let r_i be the position vector of the i'th particle w.r.t. O and r'_i be the position vector of the centre of mass w.r.t. r_i ,as shown below in the figure , then
    r_i=r'_i+R_cm                              (2)
    where R_cm is the position vector of centre of mass of the system w.r.t. O.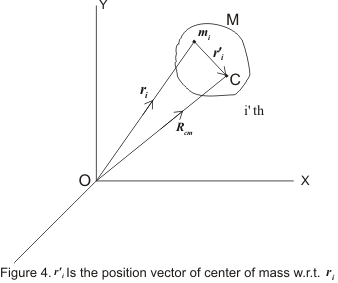
    
     
  • Differentiating equation 2 we get
    
    
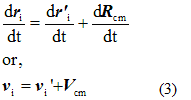
    where v_i is the velocity of i'th particle w.r.t. centre of mass and V_cm is the velocity of centre of mass of system of particle. Putting equation 3 in 1 we get,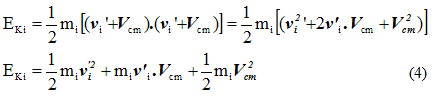
    
     
  • Sum of Kinetic energy of all the particles can be obtained from equation 4
    
    
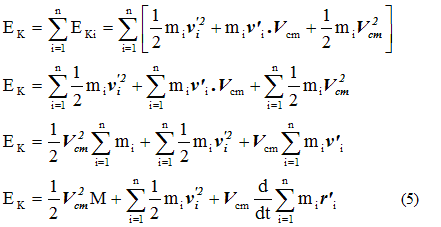
  • Now last term in above equation which is
    
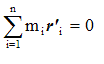
    would vanish as it defines the null vector because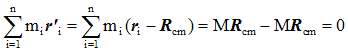
    
     
  • Therefore kinetic energy of the system of particles is,
    
    
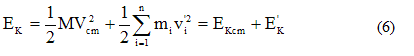
    where,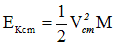
    
    
    is the kinetic energy obtained as if all the mass were concentrated at the centre of mass and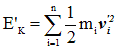
    
    
    is the kinetic energy of the system of particle w.r.t. the centre of mass.
  • Hence it is clear from equation 6 that kinetic energy of the system of particles consists of two parts: the kinetic energy obtained as if all the mass were concentrated at the centre of mass plus the kinetic energy of motion about the centre of mass.
  • If there were no external force acting on the particle system then the velocity of the centre of mass of the system will remain constant and Kinetic Energy of the system would also remain constant.

  
  
  

  (8) Two particle system and reduced mass

  
  

  
  
  • Two body problems with central forces can always be reduced to the form of one body problems.
  • Consider a system made up of two particles. For an observer in any inertial frame of refrence relative motion of these two particles can be represented by the motion of a fictitious particle.
  • The mass of this fictetious particle is known as the reduced mass of two particle system.
  • Consider a system of two particles of mass m₁ and m₂ respectively. Let O be the origin of any inertial frame of refrance and r₁ and r₂ be the position vectors of these particles at any time t w.r.t. origin O as shown bellow in the figure.
    
     
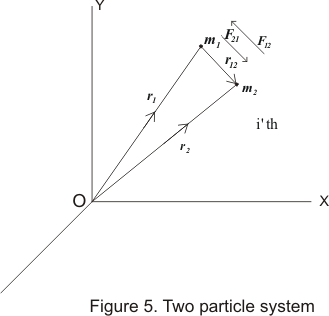
  • If no external force is acting on the system then the force acting on the system would be equal to mutual interaction between two particles. Let the force acting on m₁ due to m₂ be F₂₁ and force acting on m₂ due to m₁ be F₁₂ then equation of motion for particles m₁ and m₂ would be
    
    
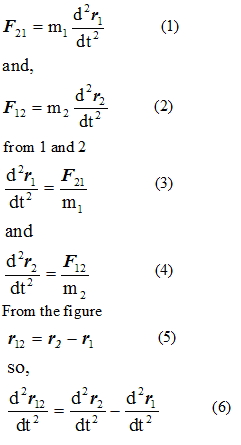
    putting 3 and 4 in 6 we get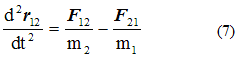
    
    
    but from Newton's first law of motion we have
    F₂₁ = -F₁₂
    then from equation 7 we have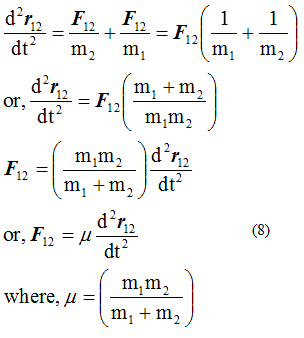
    
    
    is known as reduced mass of the system.
  • This equation 8 represents a one body problem , because it is similar to the equation of motion of single particle of mass μ at a vector distance r₁₂ from one of thr two particles, considered as the fixed centre of force.
  • Thus original problem involving two particle system has now been reduced to that of one particle system which is easier to solve then original two body problem.
    Case 1. m₁ << m₂
  • If the mass of any one particle in two particle system is very very less in comparison to other particle like in earth-satellite system then reduced mass of the system would be
    
     
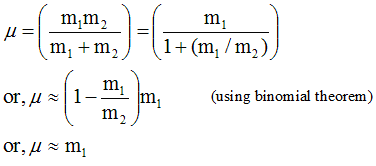
  • So the reduced mass of the two particle system would be equal to the particle having lesser mass.
    Case 2. m₁ = m₂ = m
  • If the masses o8 the particles of a two particle system are same then
    
     
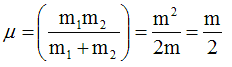
  • Hence reduced mass of the system would be equal to the one half of the mass of a single particle.

  
  (9) Linear momentum and principle of conservation of linear momentum
  

  
  
  • Product of mass and velocity of any particle is defined as the linear momentum of the particle. It is a vector quantity and its direction is same as the direction of velocity of the particle.
  • Linear momentum is represented by p. If m is the mass of the particle moving with velocity v then linear momentum of the particle would be
    p=mv
    like v , p also depends on the frame of refrance of the observer.
  • If in a many particle system m₁ , m₂ , m₃ , . . . . . . . . . . . . . , m_n are the masses and v₁ , v₂ , v₃, . . . . . . . . . . . . .,v_n are the velocities of the respective particles then total linear momentum of the system would be
    
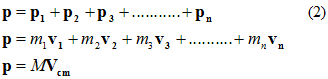
    where M is the total mass of the system and V_cm is the velocity of centre of mass of the system
  • Hence from equation 2 we came to know that total linear momentum of a many particle system is equal to the product of the total mass of the system and velocity of centre of mass of the system.
  • Differentiating equation 2 w.r.t. t we get
    
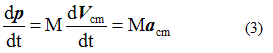
    but , Ma_cm=F_ext which is the external force acting on the system. Therefore,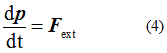
    
    like this the rate of change of momentum of a many particle system comes out to be equal to the resultant external force acting on the particle.
  • If external force acting on the system is zero then,
     
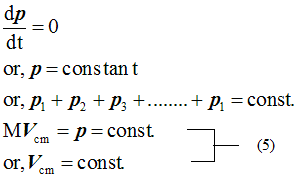
  • Hence we conclude that when resultant external force acting on any particle is zero then total linear momentum of the system remains constant. This is known as law of conservation of linear momentum.
  • Above equation 5 is equivalent to following scalar quantities
    
    Equation 6 shows the total linear momentum of the system in terms of x , y and z co-ordinates and also shows that they remain constant or conserved in absence of any externally applied force.
  • The law of conservation of linear momentum is the fundamental and exact law of nature. No violation of it has ever been found. This law has been established on the basis of Newton's law but this law holds true in the situations where Newtonian mechanics fails.

  
  (10) Centre of mass frame of refrance
  

  • If we attach an inertial frame of refrance with the centre of mass of many particle system then centre of mass in that frame of refrance would be at rest or, V_cm=0 , and such type of refrance frames are known as centre of mass frame of refrance.
  • Total linear momentum of a many particle system is zero in centre of mass frame of refrance i.e., p_cm=MV_cm=0 sinceV_cm=0. 
  • Therefore C-refrance frames are also known as zero momentum refrance frames.
  • Since in absence of any external force the centre of mass of any system moves with constant velocity in inertial frame of refrance therefore for a many particle system C-rame of refrance is an inertial frame of refrence.
  • Refrance frames connected to laboratory are known as L-frame of refrance or lebiratory frame of refrance.

  
  

  
  

  (11) Collisions

  
  

  
  
  • Collision between two particles is defined as mutual interaction between particles for a short interval of time as a result of which energy and momentum of particle changes.
  • Collision between two billiard balls or between two automobiles on road are few examples of collisions from our everyday life. Even gas atoms and molecules at room temperature keep on colliding against each other.
  • For the collision to take place , physical contact is not necessary. In cas of Rutherford alpha scattering experiment , the alpha particles are scattered due to electrostatic interaction between the alpha particles and the nucleus from a distance i.e., no physical contact occurs between the alpha particles and the nucleus.
  • Thus , in physics collision is said to have occured , if two particles physically collide with each other or even when the path of motion of one particle is affected by other.
  • In the collision of two particles law of conservation of momentum always holds true but in some collisions Kinetic energy is not always conserved.
  • Hence collisions are of two types on the basis of conservation of energy.
    (i) Perfectly elastic collision
  • Those collisions in which both momentum and kinetic energy of system are conserved are called elastic collisions for example elastic collision occurs between the molecules of a gas. This type of collision mostly takes place between the atoms, electrons and protons.
  • Characterstics of elastic collision
    (a) Total momentum is conserved.
    (b) Total energy is conserved.
    (c) Total kinetic energy is conserved.
    (d) Total mechanical energy is not converted into any other form of energy.
    (e) Forces involved during interaction are conservative in nature.
  • Consider two particles whose masses are m₁ and m₂ respectively and they collide each other with velocity u₁ and u₂and after collision their velocities become v₁ and v₂ respectively.
  • If collision between these two particles is elastic one then from law of conservation of momentum we have
    m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
    and from the law of conservation of energy we have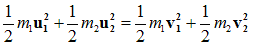
    
    (ii) Perfectly inelastic collision
  • Those collisions in which momentum of system is conserved but kinetic energy of the system is not conserved are known as inelastic collision.
  • Here in inelastic collision two bodies stick to each other after collision as a bullet hit its target and remain embedded in the target.
  • In this case some of the kinetic energy is converted into heat or is used up in in doing work in deforming bodies for example when two cars collide their metal parts are bet out of shape.
  • Characterstics of inelastic collision
    (a) Total momentum is conserved.
    (b) Total energy is conserved.
    (c) Total kinetic energy is not conserved.
    (d) A part or whole of whole mechanical energy may be converted into other forms of energy.
    (e) Some or all forces involved during interaction are non-conservative in nature.
  • Consider two particles whose masses are m₁ and m₂ respectively and they collide each other with velocity u₁ and u₂respectively. 
  • If the collision between these two particles is inelastic then these two particles would stick to each other and after collision they move with velocity v then from law of conservation of momentum we have
     
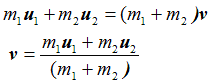
  • Kinetic energy of particles before collisions is
    
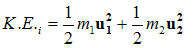
    and kinetic energy of particles after collisions is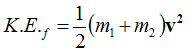
    
    by law of conserevation of energy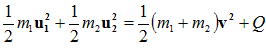
    
    where Q is the loss in kinetic energy of particles during collision.

  
  

  
  

  (12) Head on elastic collision of two particles in L-frame of refrance

  
  

  
  
  • Consider two particles whose masses are m₁ and m₂ respectively and they collide each other with velocity u₁ and u₂and after collision their velocities become v₁ and v₂ respectively.
  • Collision between these two particles is head on elastic collision. From law of conservation of momentum we have
    m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂                      (1)
    and from law of conservation of kinetic energy for elastic collision we have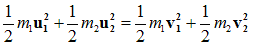
                          (2)
    rearranging equation 1 and 2 we get
    m₁(u₁-v₁)= m₂(v₂-u₂)                     (3)
    and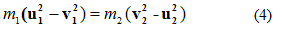
    
    dividing equation 4 by 3 we get
    u₁ + v₁ = u₂ + v₂
    u₂ - u₁ = -(v₂ - v₁)                     (5)
    where (u₂ - u₁) is the relative velocity of second particle w.r.t. first particle before collision and (v₂ - v₁) is the relative velocity of second particle w.r.t. first after collision.
  • From equation 5 we come to know taht in a perfectly elastic collision the magnitude of relative velocity remain unchanged but its direction is reversed. With the help of above equations we can find the values of v₂ and v₁ , so from equation 5
    v₁ = v₂ - u₁ + u₂                     (6)
    v₂ = v₁ + u₁ - u₂                     (7)
    Now putting the value of v₁ from equation 6 in equation 3 we get
    m₁(u₁ - v₂ + u₁ - u₂) = m₂(v₂ - u₂)
    On solving the above equation we get value of v₂ as
     
  • Similarly putting the value of v₂ from equation 7 in equation 3 we get
     
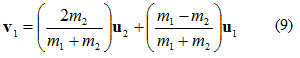
  • Total kinetic energy of particles before collision is
    
    and total K.E. of particles after collision is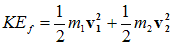
     
  • Ratio of initial and final K.E. is
     
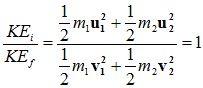
  • Special cases
    Case I: When the mass of both the particles are equal i.e., m₁ = m₂ then from equation 8 and 9 , v₂=u₁ and v₁=u₂. Thus if two bodies of equal masses suffer head on elastic collision then the particles will exchange their velocities.Exchange of momentum between two particles suffering head on elastic collision is maximum when mass of both the particles is same.
    Case II: when the target particle is at rest i,e u₂=0
    From equation (8) and (9)
    
    
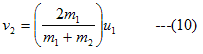
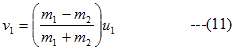
    Hence some part of the KE which is transformed into second particle would be
    
    
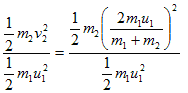
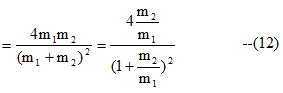
    when m₁=m₂,then in this condition v₀=0 and v₂=u₁ and part of the KE transferred would be
    =1Therefore after collisom first particle moving with initial velocity u₁ would come to rest and the second particle which was at rest would start moving with the velocity of first particle.Hence in this case when m₁=m₂ transfer of energy is 100%.if m₁ > m₂ or
    m₁ < m₂ ,then energy transformation is not 100%
    
    Case III:
    if m₂ >>>> m₁ and u₂=0 then from equation (10) and (11)
    v₁ ≅ -u₁ and v₂=0                     (13)
    For example when a ball thrown upwards collide with earth
    
    Case IV:
    if m₁ >>>> m₂ and u₂=0 then from equation (10) and (11)
    v₁ ≅ u₁ and v₂=2u₁                     (14)
    Therefore when a heavy particle collide with a very light particle at rest ,then the heavy particle keeps on moving with the same velocity and the light particle come in motion with a velocity double that of heavy particle

  (13) Head on collison of two particles in C frame of refrence
  
  

  
  
  • Consider two particles of mass m₁ and m₂ having position vectors r₁ and r₂ respectively And position vector of the CEnter of mass of the system would be R_cm
    then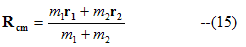
    
    Velocity of the center of mass would be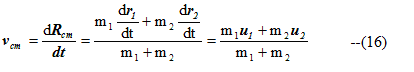
    
    Intial velocity of the m₁ w.r.t center of mass frame of refrence is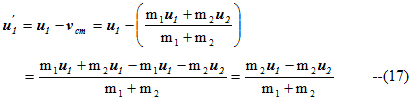
    
    Similarly Intial velocity of m₂ w.r.t center of mass frame of refrence is
    
    
  • Total linear momentum before collison in absence of external force in C frame of refrence would be
    =m₁u₁^' +m₂u₂^'
    =0
    So u₂^'=(m₁/m₂)u₁^'
    
  • If v₁^' and v₂^' are the velocites of mass m₁ and m₂ respectively after collision then by law of conservation of linear momentum
    m₁v₁^' +m₂v₂^'=0
    v₂^'=(m₁/m₂)v₁^'
    Since the collision is elastic,Kinetic energy will be conserved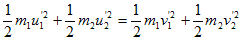
    
    
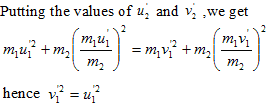
    From which |v₁^'|=|u₁^'| and |v₂^'|=|u₂^'|
    hence after collison velocities of particles remain unchanged in center of mass frame of refrence.If the collision is one dimmension then because of the collsion direction of these would be opposite to that of their intial velocites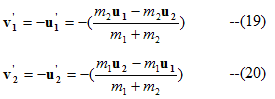
    
    
    

    (14) Head on inelastic collision of two particles which stick together

    
    A) In laboraratory frame of refrence
    
    
    
    • Consider two particles whose masses are m₁ and m₂ .Let u₁ and u₂ be the respective velocities before collision
    • Let both the particles stick together after collision and moves with the same velocity v .Then from law of conservation of linear momentum
      m₁u₁ +m₂u₂=(m₁+m₂)v
      or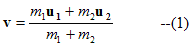
      
    • If we consider second particle to be stationary or at rest then u₂=0
      then
      m₁u₁ =(m₁+m₂)
      or
      
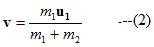
      Hence |v| < |u₁|
    • Kinetic energy before collision is
      KE₁=(1/2)m₁u₁²
      After collison KE of the system is
      KE₂=(1/2)(m₁+m₂)v²
      
      
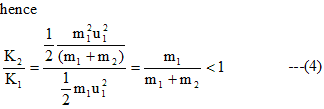
      Hence from equation we come to know that K₂ < K₁ hence energy loss would be there after thye collision of the particles
      
    
    B) In center of mass frame of refrence:-
    
    
    
    • Velocity of center of mass is
      
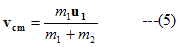
      when second particle is at rest.
      It is clear that Center of masss frame of refrence moves with velocity v_cm w.r.t laboratory frame of refrence.
      Hence velocity of particle having mass m₁ in C-frame of refrence is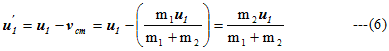
      
      And velocity of particle having mass m₂ in C-frame of refrence would be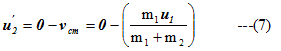
      
      
    • let v^' be the joint velocity of the particles which stick to each other after the collision in center of mass frame of refrence .So by the law of conservation of linear momentum
      (m₁+m₂)v^'=m₁u₁^' +m₂u₂^'
      Substituting the values of u₁^' and u₂^' we find
      (m₁+m₂)v^'=0
      but (m₁+m₂) can be zero so
      v^'=0
      Therefore after collision total momentum of the particles comes out to be zero.Because both the particles stick to each other after collision so the velocity of joint particle mass i.e(m₁+m₂) would be zero.Therefore in center of mass frame of refrence ,particle which stick together will remain stationary.
    
    

    (15) Deflection of an moving particle by a particle at rest during perfectly elastic collision or elastic collision in two dimension

    
    A) In labaoratory frame of refrence
    
    
    
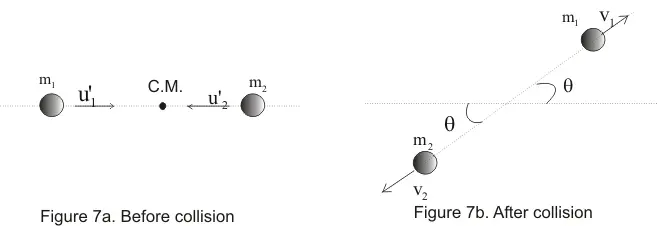
    • Let m₁ and m₂ be the two mass particle in a laboratory frame of refrence and m₁ collide with m₂ which is initailly is at rest.Let the velocity of mass m₁ before collison be u₁ and after the collison it moves with a velocity v₁ and is delfected by the angle θ₁ withs its incident direction and
      m₂ after the collision moves with the velocity v₂ and it is deflected by an angle θ₂ with its incident direction
      
       
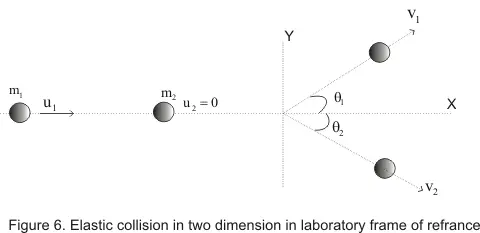
    • From law of conservation of linear momentum ,for components along x-axis
      m₁u₁=m₁v₁cosθ₁ +m₂v₂cosθ₂ ---(1)
      For components along y-axis
      0=m₁v₁sinθ₁ -m₂v₂sinθ₂ --(2)
      And from law of conservation of energy
      (1/2)m₁u₁²=(1/2)m₁v₁²+(1/2)m₂v₂² ---(3)
      
    • Analysing above equations we come to know that we have to find values of four unknown quantities v₁,v₂,θ₁,θ₂ with the help of above three equations which is impossible as we need to have atleast four equations for finding out the values of four unknown quantities .Hence this problem can be solved in C frame of refrence
      
    
    B) In center of mass frame of refrence
    
    • Velocity of the center of mass wrt to L -frame of refrence is equation
       
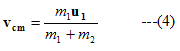
    • In C frame of refrence ,the center of mass remain stationary ,the velocity of mass m₁ before collision w.r.t C-frame of refrence is
      u₁^'=u₁ - v_cm                   (5)
      And that of mass m₂ is
      u₂^'=0 - v_cm = - v_cm                   (6)
      
      After the collision velocites of m₁ and m₂ in C-frame of refrence would be
      v₁^'=v₁ - v_cm                   (7)
      v₂^'=v₂ - v_cm                   (8)
      
      Since center of mass remains stationary in C-frame of refrence hence total momentum would be zero.Therefore the momentum of both the massed would be equal and in opposite direction
      So
      m₁u₁^' = -m₂u₂^'                     --(9)
      m₁v₁^' = -m₂v₂^'                    --(10)
      
      The above equation can be proved like this
      m₁u₁^' = m₁(u₁ - v_cm)
      
      and -m₂u₂^' = m₂v_cm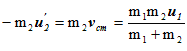
      
      
      From the above equations
      m₁u₁^' = -m₂u₂^'
      Similarly we can prove that
      
      m₁v₁^' = -m₂v₂^'
    • It is clear from the above equations that after collisions the velocity of the particles i.e v₁^',v₂^' would be in opposite direction to each other and make same angles with the direction of the intial velocities of the particles as shown fig: (b)
      
      
    • From equation (9) and (10)
      u₂^'=(m₁/m₂)u₁^'
      
      and v₂^'=(m₁/m₂)b>v₁^'
    • From the law of conservation of energy in this frame of refrence
      
       
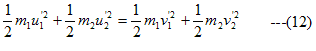
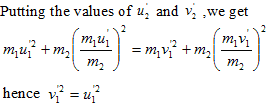
    • From which |v₁^'|=|u₁^'| and |v₂^'|=|u₂^'|.
    • From these equations we came to know that magnitude of the velocities in C-frame of refrance , when the collision is elastic, does not change but their direction could change after collision.
    
    
    Solved examples
    
    
    Question 1.A system consisting of two objects has a total momentum of (18 kgm/sec)i and its center of mass has the velocity of (3 m/s)i.One of the object has the mass 4 kg and velocity (1.5 m/s)i.The mass and velocity of the other objects are
    a. 2 kg, (6 m/s)i
    b. 2 kg, (-6 m/s)i
    c. 2 kg, (3 m/s)i
    d. 2 kg, (-3 m/s)i
    
    
    Solution 1
    Total momentum=(18 kgm/sec)i
    velocity of Center of mass=(3 m/s)i.
    Mass of one object=4 kg
    Velocity of this object=(1.5 m/s)i
    let m be the mass of other object
    And v be the velocity
    
    Now we know total momentum =Total massX velocity of center of mass
    (18 kgm/sec)i=(m+4)(3 m/s)i.
    
    or m=2 kg
    
    Now V_cm=(m₁v₁+m₂v₂)/(m₁+m₂)
    
    or
    3i=(4*1.5i + 2v)/6
    or 18i=6i+2v
    v=6i m/sec
    
    hence a is correct
    
    
    Question 2.The Position vector of the center of mass of uniform semi circular ring of radius R and Mass M whose center coincided with the origin
    a.r=(2R/π)j
    b.r=(R/π)j
    c.r=(4R/π)j
    d. none of the these
    
    Solution 2.
    
    Consider a differential element of lenght dl of the ring whose radius vector makes an angle θ with the x-axis .If the angle subtended by the length dl is dθ at the center then,dl=Rdθ
    
    Let λ be the mass per unit length
    Then mass of this element is dm=λRdθ
    
    X_cm=(1/m)∫(Rcosθ)(Rdθ) integrating from 0 and π=0
    Y_cm=(1/m)∫(Rsinθ)(Rdθ) integrating from 0 and π
    or Y_cm=2R/π
    Hence A is correct.
    
    
    Question 3.Two electrons(e) are the at the point (-a,0) and (a,0).Their mass is m.They are released from rest.The acceleration of the center of mass of the system when the electron at 4a distance apart
    a. e²/64mπε₀a²
    b. zero
    c. e²/16mπε₀a²
    d. none of the above
    
    Solution 3
    Since there are no external force on the system,center of mass remains at rest so acceleration is zero
    
    
    Question 4 An astronaut has just finished fixing a space telescope using a big instrument whose mass is one tenth as big as his mass. You realize you have no way to get back to your spaceship which is 10 meters away from you, so you throw the instrument as hard as you can in a direction away from the spaceship which causes you to move in the opposite direction, toward the spaceship. When you finally reach the space ship, how far away are you from the instrument? a.110m
    b.100m
    c.80m
    d.90
    Solution 4 In this question, we define the system to be astronaut and the instrument, and the center of mass of the system is initially at rest a distance of 10 meters from your spaceship. Since there are no external forces acting on the system and the center of mass is initially at rest, the location of the center of mass of the system can never change! If we choose the initial location of the center of mass to be at x = 0, the center of mass will always be at x = 0. The location of the center of mass of the system is determined from its definition (M_ast+M_inst)x_cm=M_astx_ast+M_instx_inst Now x_cm=0 So M_astx_ast=-M_instx_inst Since the mass of the instrument is 1/10 of mass of the astronaut, the instrument will always be ten times as far away from the center of mass as you are, and it will always be on the opposite side of the center of mass from you, So for 10 m move by astronaut, the instrument will move by 100m. Final distance of the instrument from the spaceship will be 110 m